Translating Defocus Curves from D to feet.
Posted , 9 users are following.
In trying to use defocus curves, the horizontal (D) axis can be confusing. I propose to translate that axis to feet.
Defocus curves are normalized to label the maximum acuity as zero D.
When you have an IOL placed, there is an amount of lens diopters that would make the best focus be at far distances (such as infinity). People often "target" a max sharpness on an IOL to be nearsighted by some amount. This might be expressed as -0.75 D target. When I use the term target, that is accurate for planning. Once implanted, there is a focus reality, but for convenience, I will use the word target to also mean that. Maybe there is a better word to use for both, but for what you can control, you can control your target. You cannot control your reality.
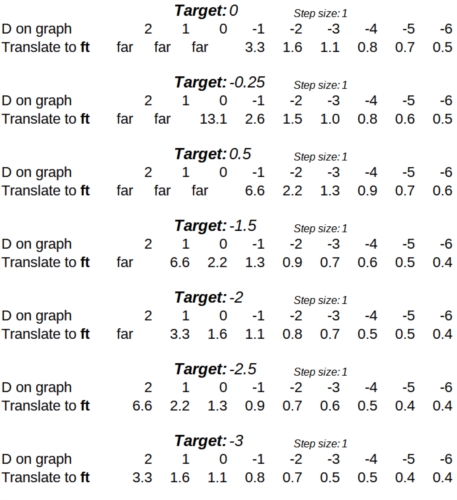
So the D-scale conversion is very much a function of the target. I have made a spreadsheet, and will post some examples for various targets.
Initially, I will choose -targets from 0 D to 3 D as targets, and provide conversions from D to ft. Example, if your target is -1.5 D, then the 0 on the horizontal axis corresponds to 2.2 ft. The -1 on the axis corresponds to 1.3 ft.
0 likes, 24 replies
trilemma
Edited
Jimluck's picture is best.
However I already expanded my spreadsheet, and it could be useful to somebody.
RonAKA trilemma
Posted
The problem with a table of values is that distances at positive D defocus values are undefined (beyond infinity). To me it is much simpler to simply divide 1 meter by the negative defocus positions to get distance in meters. The value of defocus curves to the left of the peak vision point only have meaning and value when the lens is targeted to a negative value.
trilemma RonAKA
Posted
True, but targeting (or ending up with) a negative value is pretty common, and those considering a form of monovision will be doing that.
Another thing that I think could be a revelation to some would be to have a linear distance scale on the horizontal axis. Revelation to some, but confusing to others. The use of diopters on the horizontal scale tends to devalue the part to the left. Suppose we target -1 D, I think that a graph with 10 meters on the left and 0 meters on the right could give people a different perspective.
RonAKA trilemma
Edited
Using a linear scale would not be practical. Keep in mind that 0 D is infinity for distance. If distances beyond about 12 feet were left out, then it may be of some value.
trilemma RonAKA
Posted
Yeah, 10 meters was more for shock value. Yet the story would be truth.
I agree that 12 feet is more practical, and would still tell the story.
RonAKA trilemma
Edited
I find these curves most useful in determining what the outcomes are for monofocals with the various targets including full distance. The visual acuity is in Snellen which most are familiar with and distance is in meters which is more intuitive than diopters.
.
.
And the results combined with the various targets for the near eye.
.
trilemma RonAKA
Edited
I had seen those charts, but initially found them confusing. Now they make sense. The first chart makes the most sense where they show a curve for the far (dominant) eye separately from 3 different versions of the near eye.
It took me a bit to realize that the second "combined" graph shows the use of both eyes simultaneously.
It would be nice to have a bigger study that could give smoother curves.
Thanks.
RonAKA trilemma
Posted
I think the hard reality is that defocus curves are highly smoothed already. Person to person variance can be significant. Some show error bars, but one always hopes they are on the good side of the error bar, not the bad... They are far from perfect, but really all that we have to base our decisions on.
lucy24197 trilemma
Edited
I used a slightly different approach since I was planning to have a near eye and a far eye. I made up tables that showed the distance at various visual acuities, from where the curve went from 20/40 near to 20/40 visual acuity measurements. This allowed me to look at how different lens combinations would affect each other, and what predicted results would be if I went for more distance or near vision. I could check where the "near" lens would drop below 20/32 for distance and compare the "far" lens at that distance to predict possible holes in intermediate vision.
For example, here's what I found for a -0.5D lens:
I used the distance at which vision crossed the line for 20/32 as a cutoff for "good" vision. A -0.5 lens gave me good near vision down to about 31", and my distance vision would be no worse than 20/25 from about 39" and beyond, assuming I had similar results.
For near I selected -1.75.
This predicted "good" near vision down to around 16", and the crossover between the lenses showed my intermediate vision never dropping below 20/32 with at least 20/25 vision out to around 32". (With both eyes I actually do a little better than this--I can read the J1 Jager line down to about 16", and can read the 20/20 line on the Snellen chart.)
I found making charts to see lens performance at different targets really useful for making a decision. Another thing that was VERY useful was measuring actual distances for different activities--how close is the kitchen counter for food prep, for example--to determine what visual distances you use most often--to factor into target decisions.
Note: I looked at as many defocus curves as possible and tried to include worst case results and tests with large sample sizes since near(er) vision was more important to me than distance.